領域上の記号的な偏微分方程式

バージョン11では，領域上での境界値問題の記号解および数値解を広範に渡ってサポートするようになった．

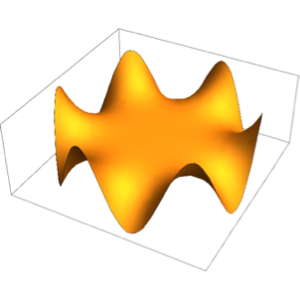
円板でのラプラス(Laplace)方程式のディリクレ(Dirichlet)問題．

In[1]:=

leqn = Laplacian[u[x, y],{x, y}] == 0;

In[2]:=

dcond = DirichletCondition[u[x, y] == Sin[6 ArcTan[y/x]], True];

In[3]:=

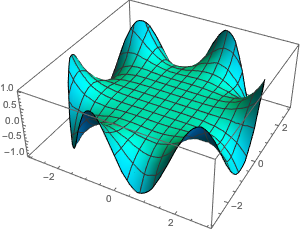
\[CapitalOmega] = Disk[{0, 0}, 3];

単位円板上の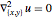をディリクレ境界条件で解く．

In[4]:=

sol = DSolveValue[{leqn, dcond}, u[x, y], {x, y} \[Element] \[CapitalOmega]]

Out[4]=

In[5]:=

Plot3D[sol, {x, y} \[Element] \[CapitalOmega], PlotRange -> All, PlotStyle -> Hue[0.5], Exclusions -> None]

Out[5]=