Transformez les structures anatomiques dans les sculptures

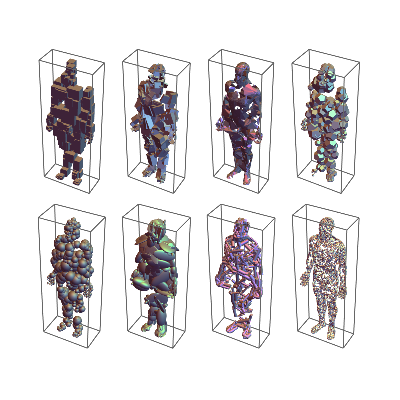
Grâce à la disponibilité de modèles 3D à haute résolution des structures anatomiques humaines, vous pouvez utiliser les puissantes fonctionnalités graphiques et de traitement des régions de la version 11 pour construire par programme des sculptures modernes basées sur l'anatomie.

L'art d'Antony Gormley peut servir d'inspiration. (http://www.antonygormley.com/sculpture/chronology).

In[1]:=

WikipediaData["Antony Gormley", "ImageList"] // Select[#, Length[Union[#]]/Length[#] &[ImageData[#][[1, 1]]] > 0.8 &] &

Out[1]=

Chargez une représentation fidèle du corps humain.

In[2]:=

human = AnatomyData[Entity["AnatomicalStructure", "Skin"], "MeshRegion"]

Out[2]=

Il est facile de construire une grande variété de sculptures en utilisant la fonctionnalité des régions.

In[3]:=

pts = MeshCoordinates[human]; nf = Nearest[pts]; color := Directive[GrayLevel[RandomReal[{0.5, 0.7}]], Specularity[RandomColor[], RandomInteger[{10, 20}]]]

In[4]:=

With[{dode = N[PolyhedronData["Dodecahedron"][[1]]]}, ballToDodecahedron[Ball[p_, r_]] := Translate[ Scale[Rotate[dode, RandomReal[{-Pi, Pi}], RandomReal[{-1, 1}, 3], {0, 0, 0}], r], p]]

In[5]:=

GraphicsGrid[ Partition[#, 4] &@{Graphics3D[{EdgeForm[], color, Table[BoundingRegion[ nf[RandomChoice[pts], RandomInteger[{100, 1000}]], "MinCuboid"], {200}]}], Graphics3D[{EdgeForm[], color, Table[BoundingRegion[ nf[RandomChoice[pts], RandomInteger[{100, 1000}]], "FastOrientedCuboid"], {250}]}], Graphics3D[{color, EdgeForm[], Table[Show[ BoundingRegion[ RandomChoice[ nf[RandomChoice[pts], RandomInteger[{100, 2000}]], RandomInteger[{4, 12}]], "MinConvexPolyhedron"]][[ 1]] /. _Directive :> {}, {600}]}], Graphics3D[{color, EdgeForm[], Table[ballToDodecahedron@ BoundingRegion[ RandomChoice[nf[RandomChoice[pts], RandomInteger[{100, 600}]], RandomInteger[{4, 12}]], "FastBall"], {300}]}], Graphics3D[{color, Table[BoundingRegion[ RandomChoice[ nf[RandomChoice[pts], RandomInteger[{100, 600}]], RandomInteger[{4, 12}]], "FastBall"], {500}]}], Graphics3D[{color, Table[ BoundingRegion[ RandomChoice[nf[RandomChoice[pts], RandomInteger[{100, 2000}]], RandomInteger[{4, 12}]], "FastEllipsoid"], {350}]}], Graphics3D[{color, EdgeForm[], Table[Cylinder[{#, nf[#, RandomInteger[{2000, 5000}]][[-1]]} &[ RandomChoice[pts]], RandomReal[{5, 25}]], {600}]}], Module[{pts2 = RandomSample[pts, 6000], nf2}, nf2 = Nearest[pts]; Graphics3D[{color, EdgeForm[], CapForm["Round"], Cylinder[{#, nf2[#, 100][[-1]]}, 6] & /@ RandomSample[pts2]}]]}, Spacings -> {-150, Automatic}, ImageSize -> Full ] // 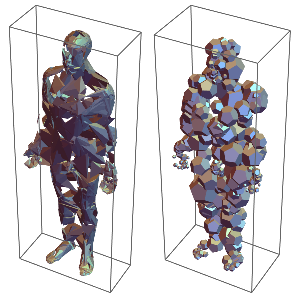Rasterize[#, "Image", ImageSize -> {400, 400}] &

Out[5]=

On peut générer une apparence de l'art classique en appliquant une transformation de rotation et d'étirement aux jambes et aux bras pour créer des sculptures angéliques.

In[6]:=

nonlinearTransform3D[expr_, g_] := Module[{gD, inv, newNormal, newVertices, newNormals}, gD[x_, y_, z_] = Compile[{x, y, z}, Evaluate[D[g[{x, y, z}], {{x, y, z}}]]]; inv[m_] := With[{im = Inverse[m]}, If[Head[im] === Inverse, m, PseudoInverse[m]]]; newNormal[{vertex_, normal_}] := Quiet[With[{m = inv[Transpose[gD[vertex]]]}, If[Sign[Det[m]] == -1, -1, 1] m.normal]]; expr /. {GraphicsComplex[vertices_, body_, a___, VertexNormals -> normals_, b___] :> (newVertices = g /@ vertices; newNormals = newNormal /@ Transpose[{vertices, normals}]; GraphicsComplex[newVertices, body, a, VertexNormals -> newNormals, b])}]

In[7]:=

doubleTwist[{x_, y_, z_}] := With[{h = 1000, w = 180, H = 1300, \[Xi] = 600, \[Kappa] = 150}, With[{\[CurlyPhi] = 6 Pi If[z > h || (z > \[Xi] && Sqrt[x^2] > w), 0, Cos[Pi/2 (h - z)/h]], \[CurlyTheta] = -1.6 Pi If[(z > \[Xi] && Sqrt[x^2] < w) || z < \[Xi], 0, Cos[Pi/2 (w - x)/w]], f = Piecewise[{{1, z > h}, {1 + (h - z)/h, z < h}}], g = If[(z > \[Xi] && Sqrt[x^2] < w) || z < \[Xi], 1, 1 + (Sqrt[x^2] - w)/\[Kappa]]}, {{g, 0, 0}, {0, Cos[\[CurlyTheta]], Sin[\[CurlyTheta]]}, {0, -Sin[\[CurlyTheta]], Cos[\[CurlyTheta]]}}.({{f Cos[\[CurlyPhi]], f Sin[\[CurlyPhi]], 0}, {-f Sin[\[CurlyPhi]], f Cos[\[CurlyPhi]], 0}, {0, 0, 1}}.{x, y, z} - {0, 0, H}) + {0, 0, H}]]

In[8]:=

smoothHuman = Entity["AnatomicalStructure", "Skin"]["Graphics3D"];

In[9]:=

Show[nonlinearTransform3D[smoothHuman, doubleTwist], ViewPoint -> {-1, -2, 0}, Method -> {"ShrinkWrap" -> True}]

Out[9]=