Physics
Expand the curriculum with exciting experiments in dynamics and gain insight into how modeling and simulation can enhance and strengthen understanding of physics and math concepts.
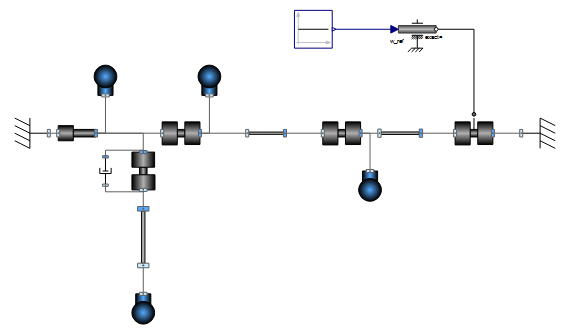
A Pendulum Dangling from a Slider-Crank
How can you use Wolfram System Modeler to model a pendulum dangling from a slider-crank?
To run this example, you'll need
The latest versions of System Modeler and Mathematica.
Please make a selection:
Get afree trial Continue
with download
The Dangling Pendulum Model
The crank wheel has a fixed translational position and a set rotational speed that drives the rest of the system. The pendulum joint has to be modeled in a certain way in order for its y position to be locked while simultaneously allowing both rotational movement and translational movement in the x direction.
The model as seen in the diagram view in Wolfram System Modeler. The pendulum joint is modeled with a prismatic joint, for the ability to lock its y position and allow movement along the x axis, and two revolute joints. Two revolute joints are needed to get the correct degrees of freedom.
Simulation and Animation
By extracting the variables describing the movement of the different mechanical parts, Wolfram System Modeler and the Wolfram Language can be combined to create an interactive visualization of the dangling pendulum.
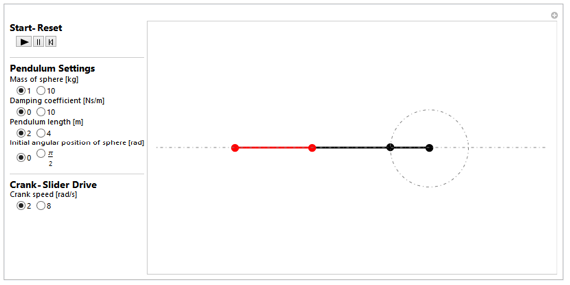
Change the settings of the dangling pendulum and see how it behaves.
Wolfram System Modeler
Try
Buy
System Modeler is available in English
and Japanese
on Windows, macOS & Linux »
Questions? Comments? Contact a Wolfram expert »