Find the Value of a European Call Option: New in Wolfram Language 11

Find the Value of a European Call Option

Find the value of a European vanilla call option if the underlying asset price and the strike price are both $100, the risk-free rate is 6%, the volatility of the underlying asset is 20%, and the maturity period is 1 year, using the Black–Scholes model.

In[1]:=

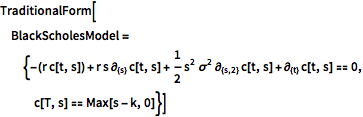
TraditionalForm[BlackScholesModel = {-(r c[t, s]) + r s \!\(
\*SubscriptBox[\(\[PartialD]\), \({s}\)]\(c[t, s]\)\) + 
     1/2 s^2 \[Sigma]^2 \!\(
\*SubscriptBox[\(\[PartialD]\), \({s, 2}\)]\(c[t, s]\)\) + \!\(
\*SubscriptBox[\(\[PartialD]\), \({t}\)]\(c[t, s]\)\) == 0, 
   c[T, s] == Max[s - k, 0]}]

Out[1]//TraditionalForm=

Solve the boundary value problem.

In[2]:=

(dsol = c[t, s] /. 
    DSolve[BlackScholesModel, c[t, s], {t, s}][[
     1]]) // TraditionalForm

Out[2]//TraditionalForm=

Compute the value of the European vanilla option.

In[3]:=

dsol /. {t -> 0, s -> 100, k -> 100, \[Sigma] -> 0.2, T -> 1, 
  r -> 0.06}

Out[3]=

Compare with the value given by FinancialDerivative.

In[4]:=

FinancialDerivative[{"European", "Call"}, {"StrikePrice" -> 100.00, 
  "Expiration" -> 1},  {"InterestRate" -> 0.06, "Volatility" -> 0.2 , 
  "CurrentPrice" -> 100}]

Out[4]=