Visually Identify Features
You can identify multiple features in a complex function from its plot.
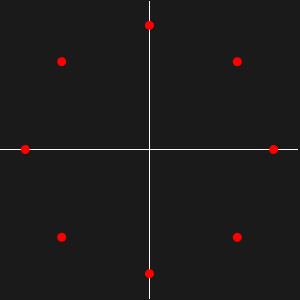
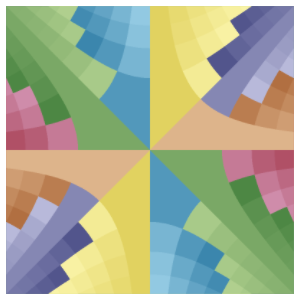
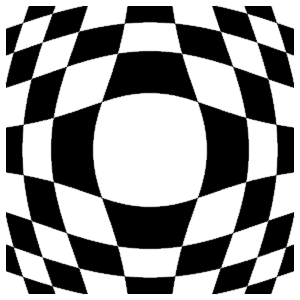
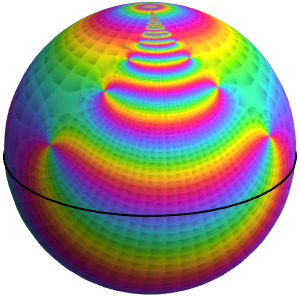
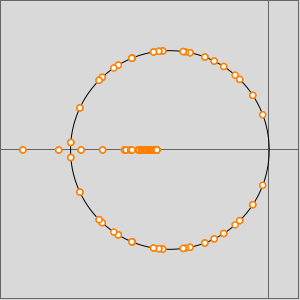
Looking at the plot of 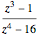 , you can easily see the zeros at the third roots of unity and the four poles at
, you can easily see the zeros at the third roots of unity and the four poles at 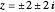 .
.
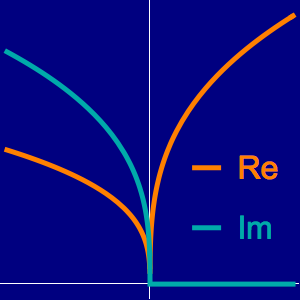
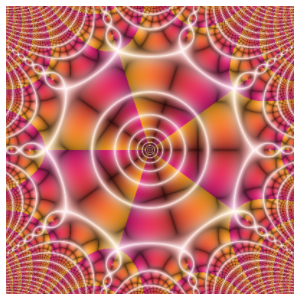
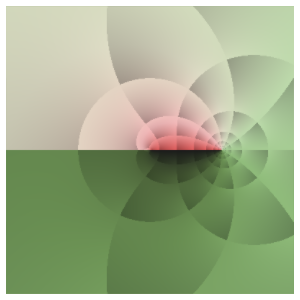
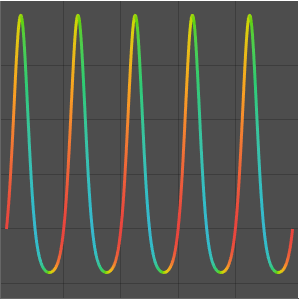
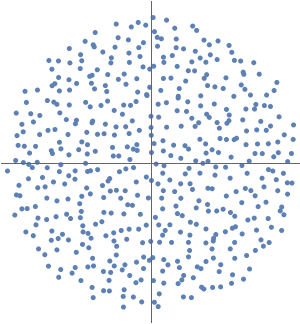
From the plot of 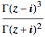 , you can note that there are zeros of order two at
, you can note that there are zeros of order two at 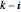 and poles of order three at
and poles of order three at 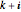 when
when 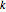 is a non-positive integer.
is a non-positive integer.
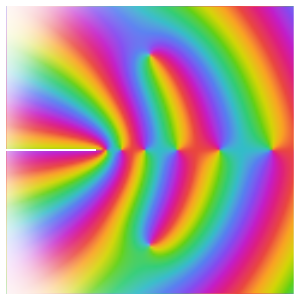
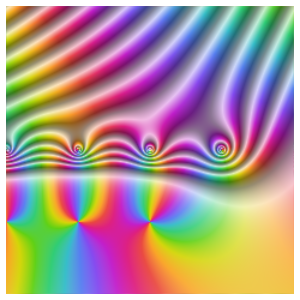
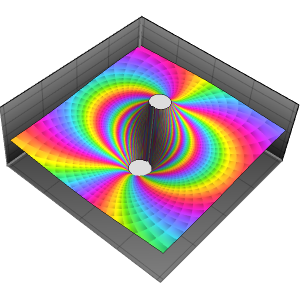
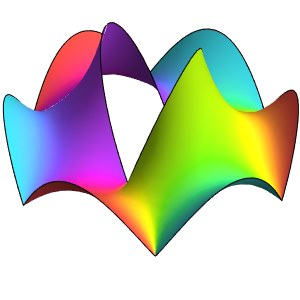
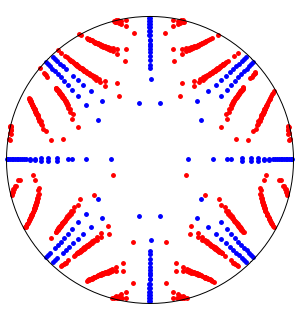
The plot of 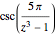 shows more complicated behavior. You can see that there are no zeros, but there is a pole of order 3 at
shows more complicated behavior. You can see that there are no zeros, but there is a pole of order 3 at 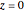 . There are also four poles of order one along each angle
. There are also four poles of order one along each angle 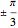 and
and 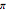 . There are also poles of order one along the angles 0 and
. There are also poles of order one along the angles 0 and 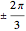 that accumulate at essential singularities at
that accumulate at essential singularities at 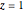 and
and 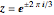 .
.