Solve a Volterra Integral Equation

Solve a Volterra integral equation using DSolveValue.

In[1]:=

eqn = y[x] == x^3 + \[Lambda] \!\( \*SubsuperscriptBox[\(\[Integral]\), \(0\), \(x\)]\(\((t - \ x)\) y[ t] \[DifferentialD]t\)\);

In[2]:=

sol = DSolveValue[eqn, y[x], x]

Out[2]=

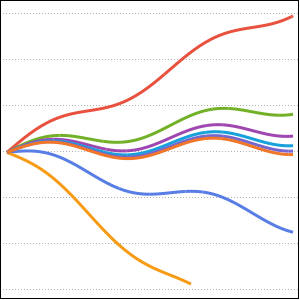
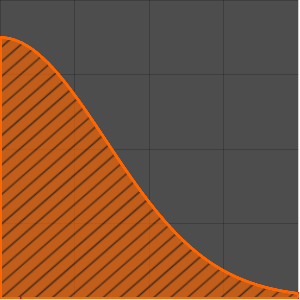
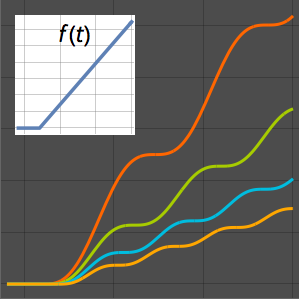
Plot the solution for different values of λ.

In[3]:=

Plot[Table[sol, {\[Lambda], 1, 3, 0.5}] // Evaluate, {x, 0, 20}]

Out[3]=

Solve a weakly singular Volterra integral equation.

In[4]:=

eqn = y[x] == x^a - \!\( \*SubsuperscriptBox[\(\[Integral]\), \(0\), \(x\)]\( \*FractionBox[\(y[t]\), SqrtBox[\(x - t\)]] \[DifferentialD]t\)\);

Use DSolveValue to obtain an expression for the solution.

In[5]:=

sol = DSolveValue[eqn, y[x], x]

Out[5]=

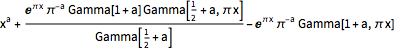
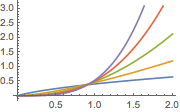
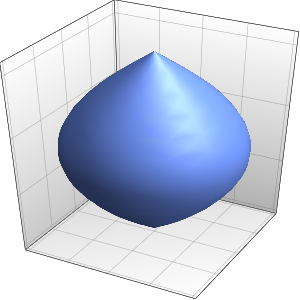
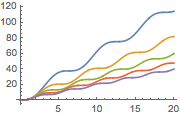
Plot the solution.

In[6]:=

Plot[Table[sol, {a, 1, 4, 0.7}] // Evaluate, {x, 0, 2}]

Out[6]=