Nonlinear Control Systems
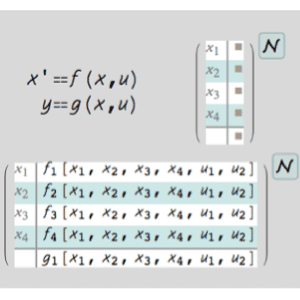
Version 10 extends its control systems capabilities to fully embrace nonlinear systems. Affine and general nonlinear systems can be exactly represented. Not only does this allow for simulation of closed-loop systems at full fidelity, but it enables a new generation of advanced design and analysis techniques. Nonlinear control design using only linear techniques and no approximation can be accomplished using exact linearization. In addition, fully nonlinear designs enable asymptotic output tracking or disturbance rejection of modeled disturbances.
- New system models for affine as well as general nonlinear systems.
- Automatic support for exact linearization of affine systems effectively enabling nonlinear control design using any linear control design technique. »
- Automatic support for Carleman linearization of general nonlinear systems to affine systems. »
- Automatic support for Taylor linearization of affine and general nonlinear systems to standard linear systems.
- Easily connect linear, affine, and nonlinear systems to simulate closed-loop systems with high fidelity.
- Full suite of standard analysis techniques (controllability, observability, vector relative orders, etc.) for affine systems.
- General state-space transformations for affine and general nonlinear systems.
- Standard canonical triangular decompositions for affine systems.
- Automatic design of full information output regulator, to compensate for modeled disturbances.
- Asymptotic output tracker to track desired output trajectories.
- Extended ability to manipulate systems, including extracting subsystem, merging systems, etc.
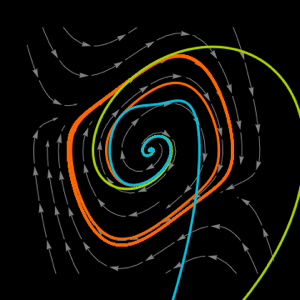
- Support for evaluating stability of nonlinear systems using circle and Popov criteria.
Related Examples
Affine Nonlinear Systems »
Examples of Affine Systems »
General Nonlinear Systems »
Example of a General Nonlinear System »
Feedback Linearization »
Estimator Design Using Feedback Linearization »
Carleman Linearization »
Evaluate a Linear Controller Design Using Nonlinear Simulation »
Related FunctionsAffineStateSpaceModel 
NonlinearStateSpaceModel FeedbackLinearize StateTransformationLinearize CarlemanLinearize LinearizingTransformationData StateSpaceTransform FullInformationOutputRegulator AsymptoticOutputTracker SystemsModelVectorRelativeOrders ControllableModelQ ObservableModelQ ControllableDecomposition ObservableDecomposition SystemsModelLinearity StateSpaceModel SystemsModelMerge StateFeedbackGains OutputResponse FeedbackSector
NonlinearStateSpaceModel FeedbackLinearize StateTransformationLinearize CarlemanLinearize LinearizingTransformationData StateSpaceTransform FullInformationOutputRegulator AsymptoticOutputTracker SystemsModelVectorRelativeOrders ControllableModelQ ObservableModelQ ControllableDecomposition ObservableDecomposition SystemsModelLinearity StateSpaceModel SystemsModelMerge StateFeedbackGains OutputResponse FeedbackSector
