Résolvez une équation intégro-différentielle
Résolvez une équation intégro-différentielle.
In[1]:=
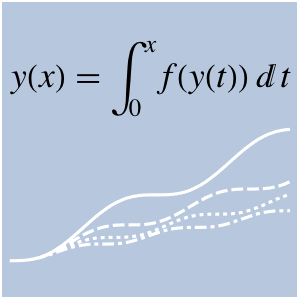
eqn = Derivative[1][y][x] == 1 + Sin[a x] + \!\(
\*SubsuperscriptBox[\(\[Integral]\), \(0\), \(x\)]\(y[
t] \[DifferentialD]t\)\);Obtenez la solution générale.
In[2]:=
sol1 = DSolveValue[eqn, y[x], x]Out[2]=
Spécifiez une condition initiale pour obtenir une solution particulière.
In[3]:=
init = y[0] == -1;In[4]:=
sol2 = DSolveValue[{eqn, init}, y[x], x]Out[4]=
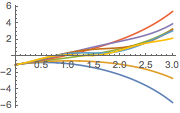
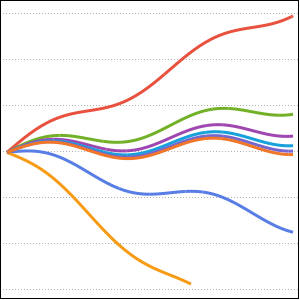
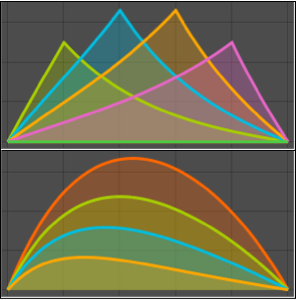
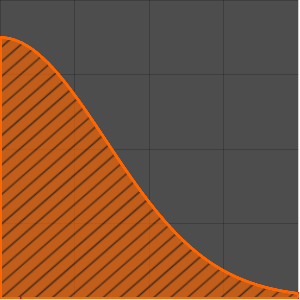
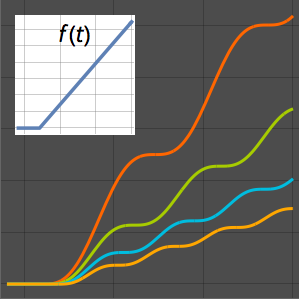
Tracez la solution.
In[5]:=
Plot[Table[sol2, {a, -1, 4, 0.7}] // Evaluate, {x, 0, 3}]Out[5]=